Binding to macromolecules

1
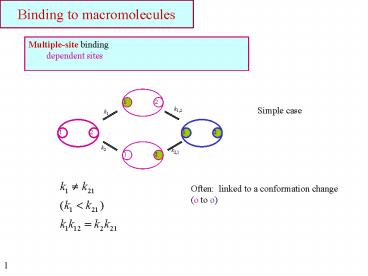
Binding to macromolecules
Multiple-site binding dependent sites
1
2
Simple case
1
2
1
2
1
2
Often linked to a conformation change (o to o)
1
2
Binding linked to conformation change
A possible
Symmetrical model has species A, AL, AL2, B, BL,
BL2
2
3
Binding to specific sites linked to conformation
change
How to evaluate a model
3
4
Thermodynamic approach to interactions of small
molecules with macromolecules
e.g., Large M and L cannot occupy same volume (L
can be a coiled H2O soluble polymer like PEG)
reference
exclusion
binding
L
L
L
L
Different L in these two situations!
constant mL
observation is n dNL/dNM ? 0 to find a general
formulation in terms ofthermodynamics or
statistical mechanics, i.e., model-free.
4
5
Thermodynamic approach to interactions of small
molecules with macromolecules
And the other way around
5
6
Ogstons models of volume exclusion
Models of volume exclusion (i) spherical
molecules. (ii) spherical and rod-like
molecules. (iii) spherical and randomly coiled
polymer molecules.
6
7
Thermodynamic approach to interactions of small
molecules with macromolecules
I wish to know how moM varies with mL (because I
need moM to analyze conformational
equilibria) The answer is
Captures binding (n gt 0) AND exclusion (n lt
0)! Model-free
7
8
Derivation
(Ideal solution of M)
8
9
Thermodynamic approach to interactions of small
molecules with macromolecules
moM varies with mL Free energy of binding
Can drive conformation change (if unequal)
9
10
Binding to macromolecules
Now consider site-specific binding as model
Check for this p.f.
10
11
Binding to macromolecules and conformation change
Equilibrium between two conformations, P and Q
depends on aL and Dn
Sensitivity of the conf. change to aL
11
12
Binding to macromolecules and conformation change
Equilibrium between two conformations, P and Q
depends on aL and Dn
? Estimate protein stability from H ion binding
kBT times the hashed area change in AQ,x AP,x
12
13
H ion binding to proteinsapproximate normal pKs
13
14
H ion binding to proteinsCauses of abnormality

• Non-specific
• Charge-charge interactions
• Linked to specific structural detail
• Proton participates in hydrogen bond as donor
• Group participates in hydrogen bond as acceptor
• (Neutral form of) side chain buried in nonpolar
  environment

All can give rise to linkage between binding and
conformation change
14
15
Charge-charge interactions global charge
distribution, spherical protein
Global treatment for a spherical model Average
net charge, Z? electrostatic potential, Fel Add
another proton dGel.static Fel ? eH
(DGºbindH)Z (DGºbindH)Z0 dGel.static
Fel wZ DpK wZ / (kBT ln 10) DGel.static
½ wZ2 ? eH
High ionic strength
Low
No interaction
w depends on ionic strength
(From Tanford)
15
16
Charge-charge interactions the continuum
dielectric model Specific charge distribution,
any shape
Continuum dielectric model Protein explicit
charges, q in a medium with low dielectric
constant, eP Solvent continuum with high
dielectric constant Poisson equation describes
electrostatic potential, Fel in such a
system (Use Poisson-Boltzmann eqn. to include
effect of ionic strength)
Solvent is polarized, which gives a
(complementary) surface charge
Coulomb energy Polarization free energy
DGel for changing one particular charge ? DpK
16
17
Continuum dielectric model details
Continuum dielectric model Numerical treatment
for specific models Charges from a MM
model Surface (Connollys) Molecular surface
17
18
A model to estimate free energy of solvation of a
protein

• Transfer the protein from vacuum to water in 4
  steps
• Turn off the charges on the protein ( Coulomb
  energy)
• Make a cavity in the water (surface free energy
  DG gt 0)
• Place the uncharged protein in the cavity
  (dispersion energy lt 0)
• Turn on the charges on the protein ( Coulomb
  energy poln energy)

18