DGLAPevolution

1
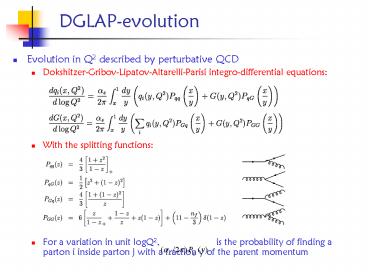
DGLAP-evolution

• Evolution in Q2 described by perturbative QCD
• Dokshitzer-Gribov-Lipatov-Altarelli-Parisi
  integro-differential equations
• With the splitting functions
• For a variation in unit logQ2,
  is the probability of finding a parton i inside
  parton j with a fraction y of the parent momentum

2
Parton distributions

• The DGLAP evolution equations determine the
  change in the parton distributions as a function
  of the scale Q2.
• No absolute prediction, only modification when Q2
  changes
• How to obtain the parton distributions in
  practice?
• Assume a parameterized functional form of the
  parton distributions at a (small) value of Q02
• A number of freeparameters
• A free starting point Q02
• Use the DGLAP evolution to calculate the value of
  the parton distribution functions at all values
  of Q2
• Small steps in Q2 approximation

3
Parton distribution sets

• Once the parton distributions are obtained for
  all values of Q2 (CPU intensive calculations!)
• Determine the structure function F2
• Compare these to the measured data of F2
• Minimizeby repeating the exercise for various
  input parameters (iterative process)
• Parameterize the set for which ?2 is minimal for
  all x and Q2 values.
• The fact that the distributions can be described
  using DGLAP evolution is a strong indication for
  the validity of QCD
• Various groups have gone through these
  calculations (also using NLO calculations) and
  published the results in the form of a computer
  program.
• Tens of distributions are available
• MRS The Durham group of Martin, Roberts and
  Stirling
• CTEQ The American group originated by Tung et al
• Botje Our NIKHEF distributions (will be extended
  to NNLO soon!)

4
Parton distributions

• Many parton distributions (PDF) are available
• Various experimental data sets included
• Order in DGLAP evolution
• Different value of strong coupling constant
• Parton distributions are universal
• They can be used to calculate cross sections at
  e.g. proton-proton colliders

5
Problems of PDF evolution

• gluon distribution functions
• grow with x (and Q2)
• large density
• cross section should scale like parton density
  (luminosity)
• violation of theoretical cross section limits?
• Froissart bound
• from optical theorem follows
• non-linear processes set in
• not only gluon splitting, but also gluon fusion!

6
Gluon Saturation

• PDFs can not increase indefinitely for low x
• non-linear effects are important for QltQS with
• high density causes perturbative behavior for
• very low x
• large nuclei
• examples
• ep at HERA?
• heavy ion collisions at RHIC?

7
Color Glass Condensate

• small x gluons generated by large x partons (e.g.
  valence quarks)
• classical color field
• high density condensate
• weak coupling
• time scales like glass
• frozen for small times
• time dilatation of fast sources
• random for long times
• very exciting new concept

8
Phenomenology of pp collisions

• Two main types of interactions
• 1) minimum-bias events (soft events).

Large distance interaction between incoming
protons where protons interact as a whole
Majority of events

• small momentum transfer (?p ? ? /?x )
• particles in final state have large longitudinal
  momentum but small transverse momentum
  (scattering at large angle is small)
  (most energy disappears down the beam-pipe)

9
Phenomenology of pp collisions
2) Hard Interactions Monochromatic proton beam
can be seen as beam of quarks and gluons with a
wide band of energy. Occasionally hard
scattering (head on) between constituents of
incoming protons occurs.
Small distances ? Large momentum transfer ?
massive particles and/or particles at large angles
10
Phenomenology of pp interactions

• Unlike ee- collisions, only a fraction of the cm
  energy available for hard interaction
• Additional experimental difficulty to reconstruct
  the kinematics

if x1 ? x2
Parton distribution functions known from DIS e-p
collisions (I.e. HERA)
11
Experimental result on large pT jets

• Experimental data on large pT jet production from
  SppS and Tevatron
• Less bias from minimum bias and trigger
• Clear jet at high Pt, assumed to be massless
• Curve a QCD prediction, O(?s3). Essentially no
  free parameters.
• Slight excess above pTgt300 GeV I.e. distances of
  10-18 m. Quark substructure?

12
Drell Yan processes

• Hadronic production of lepton pairs
• Inverse process jet-production at eg LEP
• In parton model simply weightthe process qq?ll-
  with partondistribution functions
• Good agreement between this prediction (parton
  densities from DIS!) and observations confirm
  validity of parton model approach
• First time a hadron-hadron cross section could
  be calculated from first principles
• Differential distribution as functionof lepton
  pair mass M2
• Only contribution from ?

13
QCD potential at small distance

• two-jet-events in hadron collisions
• parton-parton scattering
• QCD analog to Rutherford (Mott) scattering from
  QED
• similar dependence of cross section on scattering
  angle
• hint for 1/r behavior of potential

14
Quarkonia

• back to smaller Q2 bound states of quarks
• Coulomb-like potential at small distances?
• study heavy quark-antiquark systems
• small distance
• non-relativistic
• solve Schrödinger equation for QCD potential
• analogy to positronium
• energy level scheme of charmonium
• well described by Coulomb-like potential

15
Vector mesons - the Zweig rule

• neutral vector mesons
• light quarks
• strange quarks
• charm quarks

diagrams with unconnected quark lines are
suppressed
16
QCD potential

• Coulomb-like part at small distance
• confining potential at large distance
• linearly increasing
• can be studied in lattice QCD
• how to study bound states of light quarks?
• large radii
• relativistic
• treat in lattice QCD (still problematic) or in
  models

17
Regge trajectories

• groups of hadrons with given strangeness and
  isospin show a relation between J and M
• mesons as rotating linear quark-antiquark system
• field energy evenly distributed

18
String Model

• assume linear energy (rest mass) density
• total mass of rotating field
• with V(r) k rn

• similarly for angular momentum
• relation between J and M
• empirical law (Regge traject.)

19
Color Flux Tubes

• electrostatic field
• total flux ? constant
• strength of electric field
• potential
• color field
• self-interaction of gluons pulls field lines
  together
• total flux ?c constant
• strength of color field
• potential

20
Yo-Yo-String

• classical string
• massless quark and antiquark at the ends
• linear potential (string tension ?)
• Hamiltonian
• solution
• yo-yo motion
• enclosed area

21
String fragmentation

• fragmentation of a high energetic quark-antiquark
  system
• produces elongated string
• string breaks at eigentime ?0 producing new
  quark-antiquark pairs
• each string fragment forms a yo-yo state
  (hadron)
• length of string fragment determines mass of the
  hadron
• string fragmentation leads to ordering of space
  and momentum

22
String fragmentation II

• rapidity
• space-momentum ordering
• linear string
• plateau in rapidity distribution!

23
Phenomenology of particle production

• total multiplicity in hadron collisions
• momentum distributions
• invariant cross section
• Feynman x
• good approximation

24
Momentum distributions

• transverse momentum distributions
• approx. exponential
• mean pT
• rapidity distributions
• approx. plateau
• consistent with string fragmentation
• Feynman scaling for small xF

25
Confinement a different approach

• describe variation of coupling via
  colour-dielectric number ?e
• confinement
• QCD vacuum is a perfect colour-dielectric
• running coupling

26
Dielectric materials in EM

• ordinary materials ?r gt 1
• polarisation of medium
• perfect dielectric ?r 0
• hypothetical!
• anti-polarisation
• repulsive interaction creates spontaneous hole in
  the medium
• electrostatic potential energy balances
  deformation energy
• minimum energy
• diverges for ?r 0

27
Bag model of hadrons

• QCD vacuum is perfect colour-dielectric
• no net colour charge allowed
• only singlet states have finite energy
• quarks are confined in a bag of perturbative
  vacuum
• inside bag ?r 1
• quarks are free inside (asymptotic freedom)
• QCD vacuum extrudes color field
• analogy to superconductor (no magnetic field
  inside)
• color-electric Meissner effect

28
Hadrons QCD a quick summary

• Conserved quantum number color. Properties by
  SU(3).
• Gluons carry color, hence gluon self-interactions
• Coupling constant runs due to vacuum
  polarization
• Asymptotic freedom at high Q2 partons free
  fields inside hadrons
• Infrared slavery at low Q2 partons not observed
  outside hadrons
• DIS to probe the sub-structure of hadrons
• Scaling law experimental evidence partons
  inside proton
• Parton model successful, interpretation of (x,Q2)
• QCD induced scaling violations verified.
• Proton-proton collisions
• Folding of parton distribution functions as
  measured by DIS
• Two-jet cross section
• Drell-Yan processes
• Heavy quark (top) production/discovery