Rectangle construction

1
Rectangle construction

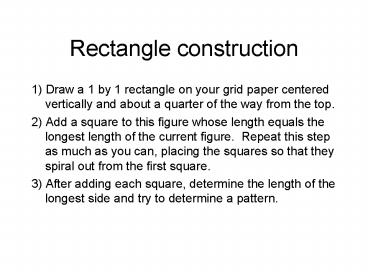
• 1) Draw a 1 by 1 rectangle on your grid paper
  centered vertically and about a quarter of the
  way from the top.
• 2) Add a square to this figure whose length
  equals the longest length of the current figure.
  Repeat this step as much as you can, placing the
  squares so that they spiral out from the first
  square.
• 3) After adding each square, determine the length
  of the longest side and try to determine a
  pattern.

2
Fibonacci sequence

• What rule governs this sequence?
• History named for Fibonaccis problem in the
  book Liber abaci published in 1202
• A certain man put a pair of rabbits in a place
  surrounded on all sides by a wall. How many pairs
  of rabbits can be produced from that pair in a
  year if it is supposed that every month each pair
  begets a new pair which from the second month on
  becomes productive?

3
Connections with nature

• Flower petals - many varieties of flowers have a
  number of petals equal to a Fibonacci number
• Pinecone spirals

4
Finding ratios

• Compute F1 through F20.
• Compute the ratios Fn/Fn-1 for values of n
  ranging from 2 to 20.
• what patterns do you observe?
• are the ratios converging?
• Call this number of convergence F and find a
  formula.

5
Solving equations

• A reminder to solve
• use the quadratic formula
• Use this to find an expression for F.

6
The Golden Mean

• F is called
• the golden mean
• the golden ratio
• the divine proportion
• If you break a bar into two pieces so that the
  ratio of the long piece to the short equals the
  ratio of the whole piece to the long, both ratios
  are F.

7
The Golden Rectangle

• A rectangle is golden if the ratio of its long
  side to its short side is F.

Source Mark Frietags site Phi That Golden
Number
8
Constructing a Golden Rectangle

• Draw a square and bisect the bottom side.
• Draw a line from that side to the top right
  corner.
• Draw a line with that same length extending from
  the bisection point parallel to the bottom side.
• Complete the rectangle. (You can measure the
  sides to check the ratio.)

9
Why does this work?

• Reminder given a right triangle with short sides
  a and b and hypotenuse c, we have the Pythagorean
  Theorem
• Use this to find the ratio.

10
A beautiful result

• Given a golden rectangle, if you divide it into a
  square and a rectangle, the rectangle is golden.

A example (with a logarithmic spiral) from Mark
Frietags site Phi That Golden Number
11
Question for Friday

• Clearly, F has some important mathematical
  properties.
• Does it have important aesthetic properties?