Kein Folientitel - PowerPoint PPT Presentation
1 / 1
Title:
Kein Folientitel
Description:
Current multiple suppression techniques may be divided into three general ... This profile was collected in the Shetland-Faeroes basin and contains a strong ... – PowerPoint PPT presentation
Number of Views:49
Avg rating:3.0/5.0
Title: Kein Folientitel
1
Removing multiples from the wide-angle
wavefield Immo Trinks
trinks_at_esc.cam.ac.uk
- The possible methods
- Current multiple suppression techniques may be
divided into three general categories - NMO based
methods, deconvolution and wave-equation
demultiple methods. - Normal moveout (NMO) based methods
- Simple stacking
- F-k filtering after NMO correction with
intermediate NMO-velocity - Parabolic and hyperbolic Radon transform
- ? problem automatic definition of the
reject/pass zone of filter - Karhunen-Loéve transform eigenvalue
decomposition after NMO - correction with multiple velocity
- ? greatest coherency for the multiples
- ? the highest eigenvalues correspond to the
flattened multiples - ? reconstruction of the data omitting the
highest eigenvalues - Adaptive minimum variance unbiased (MVU)
beamforming - ? wavefield decomposition multichannel filter
- ? extracts coherent signals without distortion
On several occasions during the past several
years, various geophysicists have expressed, in
private discussion, doubt regarding the existence
of multiple reflections or at least their
recordability over background noise. Dix, C. H.,
1948, The existence of multiple reflections,
Geophysics XIII, p. 49
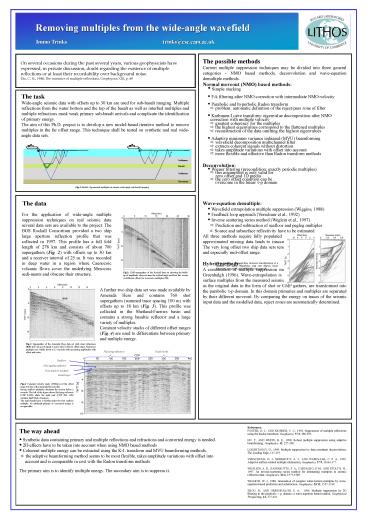
The task Wide-angle seismic data with offsets up
to 30 km are used for sub-basalt imaging.
Multiple reflections from the water bottom and
the top of the basalt as well as interbed
multiples and multiple refractions mask weak
primary sub-basalt arrivals and complicate the
identification of primary energy. The aim of this
Ph.D. project is to develop a new model-based
iterative method to remove multiples in the far
offset range. This technique shall be tested on
synthetic and real wide-angle data sets.
Sediment
Basalt
Sediment
Basement
Fig.1 Model of generated multiples in seismic
wide-angle sub-basalt imaging
The data
For the application of wide-angle multiple
suppression techniques on real seismic data
several data sets are available to the project.
The BGS Rockall Consortium provided a two ship
large aperture reflection profile that was
collected in 1997. This profile has a full fold
length of 278 km and consists of about 700
supergathers (Fig. 2) with offsets up to 30 km
and a receiver interval of 25 m. It was recorded
in deep water in a region where Caenozoic
volcanic flows cover the underlying Mesozoic
sedi-ments and obscure their structure.
(M)
(M)
Fig.5 Linear ?-p (intercept-time slowness)
transformation of a synthetic shot gather.
Hyperbolae map onto ellipses, linear refractions
onto points, and multiples become periodic.
(M)
Fig.2 CMP-supergather of the Rockall data set
showing the build-up of amplitude observed near
the critical angle and how this occurs on
different offsets for successive multiples (M)
A further two ship data set was made available by
Amerada Hess and contains 760 shot supergathers
(summed trace spacing 100 m) with offsets up to
18 km (Fig. 3). This profile was collected in the
Shetland-Faeroes basin and contains a strong
basaltic reflector and a large variety of
multiples. Constant velocity stacks of different
offset ranges (Fig. 4) are used to differentiate
between primary and multiple energy.
(Ref)
Fig.3 Supergather of the Amerada Hess data set
with clear refractions (Ref) and very good signal
to noise ratio in the far offset range. Numerous
multiples are visible down to 15 seconds with
increasing amplitudes with offset and order.
Fault blocks
Flat lying reflectors
Seafloor
Thin dipping reflector
First seafloor multiple
Basalt layer
Fig.4 Constant velocity stack (1500m/s) of the
offset range 0-6 km of the Amerada Hess
profile. Strong seafloor multiples dominate the
section below 2 seconds. The left of the figure
shows flat lying reflectors (CDP 0-200) while the
right part (CDP 200- 400) contains fault block
structures. The main basalt layer is hidden under
the first seafloor multiple. No subbasalt primary
or converted energy is recognisable.
- The way ahead
- Synthetic data containing primary and multiple
reflections and refractions and converted energy
is needed. - 2D effects have to be taken into account when
using NMO based methods - Coherent multiple energy can be extracted using
the K-L transform and MVU beamforming methods. - ? the adaptive beamforming method seems to be
most flexible, takes amplitude variations with
offset into - account and is comparable in cost with the
Radon transform methods - The primary aim is to identify multiple energy.
The secondary aim is to suppress it.
References FOSTER, D. J., AND MOSHER, C. C.,
1992. Suppression of multiple reflections using
the Radon transform, Geophysics, 57/3,
386-395. HU, T., AND WHITE, R. E., 1998. Robust
multiple suppression using adaptive beamforming,
Geophysics, 42, 227-248. LOKSHTANOV, D., 1999.
Multiple suppression by data-consistent
deconvolution, The Leading Edge,
115-119. VERSCHUUR, D. J., BERKHOUT, A. J., AND
WAPENAAR, C. P. A., 1992. Adaptive
surface-related multiple elimination, Geophysics,
57/9, 1166-1177. WEGLEIN, A. B., GASPAROTTO, F.
A., CARVALHO, P. M., AND STOLT R. H., 1997. An
inverse-scattering series method for attenuating
multiples in seismic reflection data, Geophysics,
62/6, 1975-1989. WIGGINS, W. J., 1988.
Attenuation of complex water-bottom multiples by
wave-equation-based prediction and substraction,
Geophysics, 53/12, 1527-1539. ZHOU, B., AND
GREENHALGH, S. A., 1996. Multiple suppression by
2D filtering in the parabolic ? -p domain a
wave-equation based method, Geophysical
Prospecting, 44, 375-401.